LABORATORY WORK № 2

MAGNETIC CIRCUITS OF TRANSFORMERS

2.1. Objectives

1.       Get acquainted with operation and properties of single phase transformer;

2.       Acquire skills to measure transformer parameters and operating characteristics.

 

2.2. Tasks

1.       Analyze connection of measuring devices and parameters to be measured;

2.       Obtain experimentally operating characteristics and characteristic parameters of single phase transformer.

3.       Analyze the primary winding current shape variation at load change from zero to the rated one.

 

2.3.         Magnetic circuits of transformers

A magnetic core provides a path for magnetic flux and is an integral part of transformers and electric machines. The core material of the magnetic circuit (constituting a trans-former or an electric machine) is generally of such a material that the variation of B with His depicted by the saturation curve shown in Fig. 2.1.

 

 

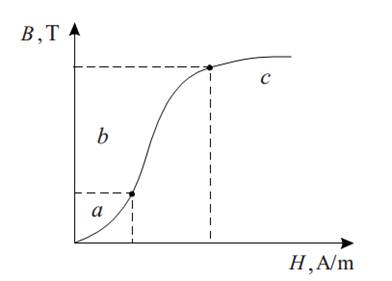
Fig. 2.1. Saturation curve of magnetic materials

 

The slope of the curve depends upon the operating flux density as classified in regions a, b, c. For region b that has a constant slope we may write:

 

 

Core losses

Consider the magnetic circuit of the transformer shown in Fig. 1.1. Alternating current develops alternating magnetic moving force (MMF) in magnetic circuit. Then B–H curve takes the form shown in Fig. 2.2.

This loop is known as the hysteresis loop and the area within the loop is proportional to the energy loss (as heat) per cycle. This energy loss is known as hysteresis loss.

Eddy current loss, the loss due to eddy currents induced in the core materials of a magnetic circuit excited by AC MMF, is another feature of an AC-operated magnetic circuit. The power losses due to hysteresis and eddy currents collectively known as core losses or iron losses are approximated by:

 

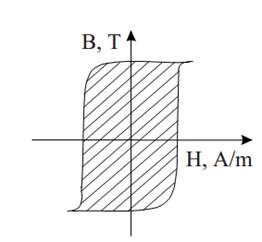
Fig. 2.2. Hysteresis loop

 

Eddy current loss:

 

 

Hysteresis loss:

 

 

The hysteresis loss component of the core loss is reduced using «good quality» electrical steel (having a narrow hysteresis loop) for the core material.

The eddy current loss is reduced making the core of laminations or thin sheets with very thin layers of insulation between the laminations. The laminations are oriented parallel to the direction of flux. Lamination of a core increases its cross-sectional area and hence its volume. The ratio of the volume actually occupied by the magnetic material to the total volume of the core is called the stacking factor.

 

Phasor Diagram of linear transformer

A linear transformer has no core or a non-ferromagnetic core or ferromagnetic non-saturated core (Fig. 2.3).

 

 

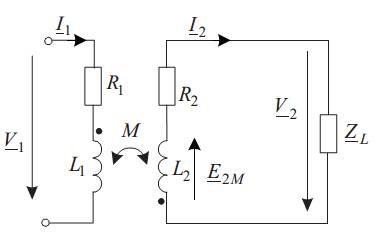
Fig. 2.3. Linear transformer

This circuit is used for the analysis of characteristics of trans-formers. The ideal transformer is close to an actual transformer with a large number of turns and with a magnetic core of high permeability. According to the system of equations eddy current loss and hysteresis loss the equivalent circuit of the linear transformer looks like shown in Fig. 2.4.

 

 

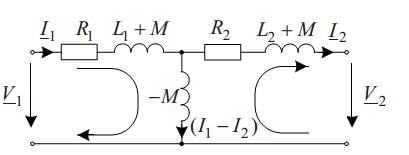
Fig. 2.4. Equivalent circuit of a linear transformer

 

The equivalent circuit in Fig. 2.4 includes the series resistance of coil  R₁ and X₁, the leakage reactance, which arises from leakage fluxes as well as magnetizing reactance:

 

 

across which terminal voltage V₁ is shown.

The hysteresis and eddy current losses are reflected by the resistance R_c in parallel with reactance:

 

 

so that voltage V₁ appears across R_c also, the core losses being directly dependent on V₁ is used for accounting of core losses. Voltage regulation is a measure of the change in the terminal voltage of the transformer with a load.

 

 

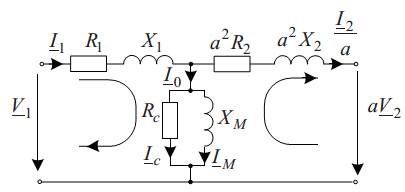
Fig. 2.5. Equivalent circuit of a transformer

 

 

 

2.4. Method of testing

1.       Get acquainted with experimental circuit in Fig. 2.3, 2.4, 2.5 and measuring devices as well as the purpose of those.

2.       Check the switch of supply voltage to be in turned off position.

3.       Set the slider of rheostat to get the greatest value of resistance.

4.       Check the scale limits of measuring devices: do they fit values of the measured parameters: voltage 40V and cur-rent 5A.

5.       After fulfilling requirements of 1.1–1.3 items, connect the experimental circuit to supply voltage.

6.       Turn on supply voltage by automatic switch QF1.

7.       Test of transformer at no-load.

8.       Disconnect one wire of load rheostat to set up secondary current, equal to zero.

9.       Write readings of V_1r , I₁₀,  and V₂₀ into Table.

10.  Redraw from measuring device METREL or computer screen the curves of voltage and current and calculate percentage of the third harmonic in the primary current.

11.  According to the experimental data calculate turns ratio and core losses.

12.  Short-circuit experiment.

13.  Set up slider of autotransformer in position to get the sup-ply voltage equal to zero. Check if the measuring device METREL shows voltage equal to zero.

14.  Connect the wire, being disconnected at no-load test and slider of the load rheostat setup in position, giving load resistane, equal to zero.

15.  By autotransformer AT slowly increase primary voltage while the secondary current will reach its rated value 5A.

16.  Write in the table readings V_1Sh, I_1Sh, P_1Sh and I_2Sh .

17.  Redraw from measuring device METREL or computer screen the curves of voltage and current and calculate percentage of the third harmonic in the primary current.

18.  After short circuit experiment rebuild experimental circuit: slider of load rheostat set up in position of the greatest resistance and by autotransformer set up the rated volt-age 220V.

19.  Performance characteristics.

20.  Calculate power and efficiency for each load value and write data to the Table.

21.  Observe the variation of primary current shape and harmonic composition during loading transformer.

22.  Turn off the voltage by automatic switch QF after completing the experiment.

 

2.5. Content of report

1.       Task of the work and experimental circuit.

2.       Experimental data of transformer parameters at no load, short circuit and voltage regulation.

3.       Voltage regulation characteristics, plotted in the same figure: .

4.       Core losses and heat losses, turns ratio, relative short circuit voltage and relative voltage increment.

5.       Shapes of primary current for three load current values.

6.       Conclusions about: shape of primary current and its harmonical composition, change of voltage with load and its relative increment, variation of efficiency and power factor with load, core and heat losses – how many percents they constitute from the rated transformer power.

 

2.6. Control questions

1.       What changes in the loaded transformer comparing with transformer at no-load?

2.       Why does the secondary voltage reduce with increasing load?

3.       Plot the equivalent circuit of transformer and explain the physical meaning of circuit elements.

4.       Explain, what will happen with the transformer if there will be an air gap in its core.

5.       Explain, what will happen with the transformer if its core is made of solid iron.

6.       Why power factor  is not equal to zero at the load current equal to zero?

7.       What are the main reasons of primary current distortion at operation on no-load?

8.       What power does the transformer characterize?

9.       What losses do appear in the transformer?

10.  On what factors do the core losses depend?